19 An Intertwined Issue

© Zyanya Santuario, MATH+

Play around with the cogs: Rotating Cogwheels – Mathekalender

Author: Lukas Protz (TU Berlin, MATH+)

Challenge

It is dark and dusty. What else does one expect from Santa’s attic at the North pole? Only equipped with a small flashlight, the eager elf Eifi wanted to clean the attic of Santa’s workshop so that there was more room for all the presents to be stored. To his surprise, the attic is full of little treasure chests sealed by a weird mechanism. Eifi is wondering whether Santa is hiding something from the elves, and eager as he is, he tries to open the chests. He discovers that the mechanism works as follows:

• On the lid of each chest are three cogs. The number of teeth on each cog can be equal or different from the numbers of teeth of the other two cogs.
• When looking directly down at the cogs, one tooth of each gear always points straight up.
• Additionally, there are three buttons on the box. When a button is pressed, two of the cogs rotate simultaneously. The rotation always occurs clockwise, moving each cog forward by one tooth. Buttons cannot be pressed simultaneously but only one after another.
• The cogs are assigned to the buttons as follows:
  • Button 1 rotates cogs 2 and 3
  • Button 2 rotates cogs 1 and 3
  • Button 3 rotates cogs 1 and 2
• The box opens when all three marked teeth point upwards.

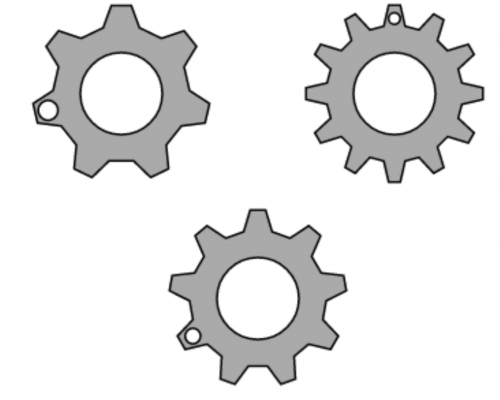
Figure 1: Example of a starting configuration of three cogs. Only the marked tooth of the upper right cog is facing upwards.

Unfortunately, Eifi has no strategy when trying to unlock the chests and only manages to open a few chests. But that does not stop Eifi from continuing his quest to uncover all the secrets that Santa may hide in them. If only there were a universal manual on how to open all the chests…

Can you help Eifi? For which combinations of three cogs with a number of teeth a,~b, and c is it possible to open the chest, independent of the starting position of each cog?

Remark: The question asks for the largest set of triples a,~b and c or equivalently for the smallest sufficient restriction on a,~b and c.

Possible Answers

1. It is never possible.
2. a,~b and c are pairwise coprime (share no common factor).
3. Two of the numbersa,~b and c are coprime.
4. a,~b and c are not divisible by 2.
5. Not all of the numbers a,~b and c are divisible by 2.
6. a,~b and c are all divisible by 2.
7. a,~b and c are not divisible by 3.
8. Not all of the numbers a,~b and c are divisible by 3.
9. a,~b and c are all divisible by 3.
10. None of the other possible answers apply.