
© Ivana Martic, MATH+
Authors: Rudolf Straube (Heinrich-Hertz-Gymnasium Berlin) and Arthur Straube (ZIB)
Project: AA1-18
Challenge
The elves Bob and Sieglinde were sitting happily playing chess on Advent Day. Suddenly the clock struck, heralding their shift at the Christmas factory. Their task in the Christmas factory is to coat cookies, consisting of 10 square pieces, of the rectangular shapes 1 \times 10 and 2 \times 5 with glaze (see figure 1).
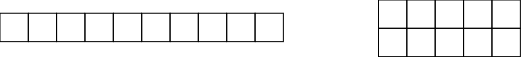
Figure 1
To ensure that the cookies remain unique in their decoration, Bob and Sieglinde coat pieces of the cookies with glaze according to the “Christmas chess principle”. Like chess kings hitting the up to eight squares around them on a chess board, they only apply the glaze to the individual squares in such a way that no two squares covered with glaze touch each other on an edge or corner. At the same time, however, there must be no space left for another glazed square on the entire cookie. Optimization is not an issue here. It is therefore quite possible that in one arrangement more and in another fewer glaze squares would have fitted on the entire rectangular cookie.
How many different ways are there to glaze the cookies in the shapes 1 \times 10 and 2 \times 5? The answer must be given in pairs of numbers (M, N), where M stands for the shape 1 \times 10 and N for the shape 2 \times 5.
Note: Consider only the final configurations as different. Moreover, configurations that can be transformed into each other by rotating the cookie are also considered as different.
Possible Answers:
- (9, 12)
- (12, 12)
- (12, 16)
- (12, 20)
- (12, 24)
- (12, 36)
- (16, 16)
- (16, 20)
- (16, 24)
- (16, 36)